CONONICAL FORMS (SOP AND POS)

 CONONICAL FORMS

The use of switching devices like transistors give rise to a special case of the Boolean algebra called as switching algebra. In switching algebra, all the variables assume one of the two values which are 0 and 1.

In Boolean algebra, 0 is used to represent the ‘open’ state or ‘false’ state of logic gate. Similarly, 1 is used to represent the ‘closed’ state or ‘true’ state of logic gate.

A Boolean expression is an expression which consists of variables, constants (0-false and 1-true) and logical operators which results in true or false.

TWO TYPE OF CONONICAL FORMS;

A Boolean function is an algebraic form of Boolean expression. A Boolean function of n-variables is represented by f(x1, x2, x3….xn). By using Boolean laws and theorems, we can simplify the Boolean functions of digital circuits. A brief note of different ways of representing a Boolean function is shown below.

• Sum-of-Products (SOP) Form
• Product-of-sums (POS) form
• Canonical forms

There are two types of canonical forms:

• Sum-of-min terms or Canonical SOP
• Product-of- max terms or Canonical POS

Boolean functions can be represented by using NAND gates and also by using K-map (Karnaugh map) method. We can standardize the Boolean expressions by using by two standard forms.

SOP form – Sum Of Products form

POS form – Product Of Sums form

Standardization of Boolean equations will make the implementation, evolution and simplification easier and more systematic.

CONICAL FORM (STANDARD SOP AND POS FORM);  

  

Any Boolean function that is expressed as a sum of minterms or as a product of max terms is said to be in its “canonical form”.

It mainly involves in two Boolean terms, “minterms” and “maxterms”.

When the SOP form of a Boolean expression is in canonical form, then each of its product term is called ‘minterm’. So, the canonical form of sum of products function is also known as “minterm canonical form” or Sum-of-minterms or standard canonical SOP form.

Similarly, when the POS form of a Boolean expression is in canonical form, then each of its sum term is called ‘maxterm’. So, the canonical form of product of sums function is also known as “maxterm canonical form or Product-of sum or standard canonical POS form”.

MIN TERMS;

A minterm is defined as the product term of n variables, in which each of the n variables will appear once either in its complemented or un-complemented form. The min term is denoted as mi where i is in the range of 0 ≤ i < 2ⁿ.

A variable is in complemented form, if its value is assigned to 0, and the variable is un-complimented form, if its value is assigned to 1.

For a 2-variable (x and y) Boolean function, the possible minterms are:

x’y’, x’y, xy’ and xy.

For a 3-variable (x, y and z) Boolean function, the possible minterms are:

x’y’z’, x’y’z, x’yz’, x’yz, xy’z’, xy’z, xyz’ and xyz.

• 1 – Minterms = minterms for which the function F = 1.
• 0 – Minterms = minterms for which the function F = 0.

Any Boolean function can be expressed as the sum (OR) of its 1- min terms. The representation of the equation will be

• F(list of variables) = Σ(list of 1-min term indices)

Ex: F (x, y, z) = Σ (3, 5, 6, 7)

The inverse of the function can be expressed as a sum (OR) of its 0- min terms. The representation of the equation will be

• F(list of variables) = Σ(list of 0-min term indices)

Ex: F’ (x, y, z) = Σ (0,1, 2, 4)

Examples of canonical form of sum of products expressions (min term canonical form):

i) Z = XY + XZ′

ii) F = XYZ′ + X′YZ + X′YZ′ + XY′Z + XYZ

In standard SOP form, the maximum possible product terms for n number of variables are given by 2ⁿ. So, for 2 variable equations, the product terms are 22 = 4. Similarly, for 3 variable equations, the product terms are 23 = 8.

MAX TERMS;

A max term is defined as the product of n variables, within the range of 0 ≤ i < 2ⁿ. The max term is denoted as Mi. In max term, each variable is complimented, if its value is assigned to 1, and each variable is un-complimented if its value is assigned to 0.

For a 2-variable (x and y) Boolean function, the possible max terms are:

x + y, x + y’, x’ + y and x’ + y’.

For a 3-variable (x, y and z) Boolean function, the possible maxterms are:

x + y + z, x + y + z’, x + y’ + z, x + y’ + z’, x’ + y + z, x’ + y + z’, x’ + y’ + z and x’ + y’ + z’.

• 1 – Max terms = max terms for which the function F = 1.
• 0 – max terms = max terms for which the function F = 0.

Any Boolean function can be expressed the product (AND) of its 0 – max terms. The representation of the equation will be

• F(list of variables) = Π (list of 0-max term indices)

Ex: F (x, y, z) = Π (0, 1, 2, 4)

The inverse of the function can be expressed as a product (AND) of its 1 – max terms. The representation of the equation will be

• F(list of variables) = Π (list of 1-max term indices)

Ex: F’ (x, y, z) = Π (3, 5, 6, 7)

Examples of canonical form of product of sums expressions (max term canonical form):

i. Z = (X + Y) (X + Y′)

ii. F = (X′ + Y + Z′) (X′ + Y + Z) (X′ + Y′ + Z′)

In standard POS form, the maximum possible sum terms for n number of variables are given by 2ⁿ. So, for 2 variable equations, the sum terms are 22 = 4. Similarly, for 3 variable equations, the sum terms are 23 = 8.

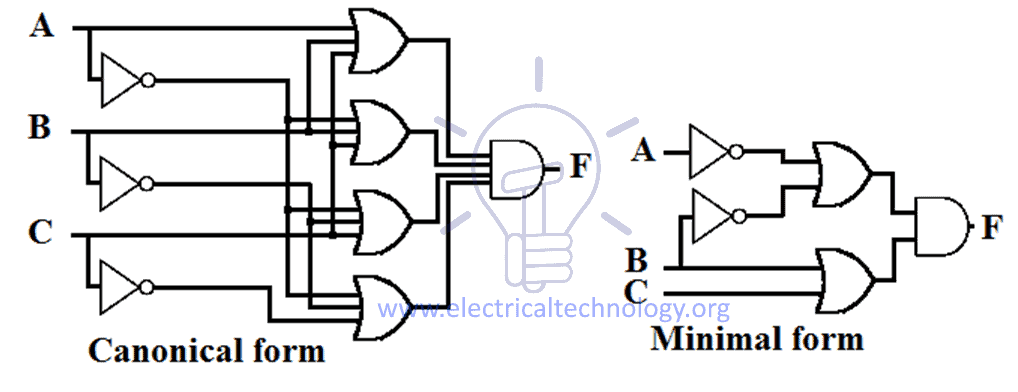
Table for 2n min terms and 2n max terms

The below table will make you understand about the representation of the mean terms and max terms of 3 variables.

TABLE;

                                                                  

1.SUM OF PRODUCTS (SOP);

The sum-of-products (SOP) form is a method (or form) of simplifying the Boolean expressions of logic gates. In this SOP form of Boolean function representation, the variables are operated by AND (product) to form a product term and all these product terms are ORed (summed or added) together to get the final function

A sum-of-products form can be formed by adding (or summing) two or more product terms using a Boolean addition operation. Here the product terms are defined by using the AND operation and the sum term is defined by using OR operation.

The sum-of-products form is also called as Disjunctive Normal Form as the product terms are ORed together and Disjunction operation is logical OR. Sum-of-products form is also called as Standard SOP.

SOP form representation is most suitable to use them in FPGA (Field Programmable Gate Arrays).

EXAMPLES;

AB + ABC + CDE

(AB) ̅ + ABC + CD E ̅

SOP form can be obtained by

• Writing an AND term for each input combination, which produces HIGH output.
• Writing the input variables if the value is 1, and write the complement of the variable if its value is 0.
• OR the AND terms to obtain the output function.

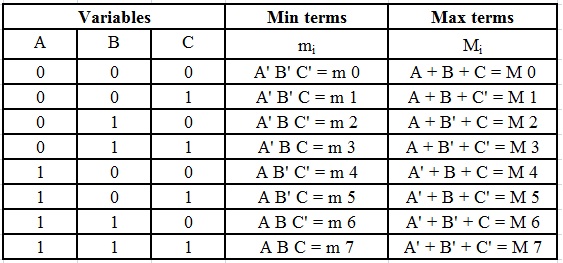
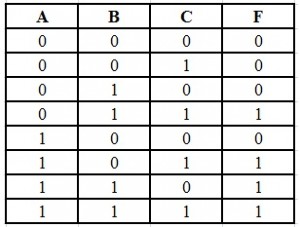
Ex: Boolean expression for majority function F = A’BC + AB’C + ABC ‘ + ABC

TRUTH TABLE;

Now write the input variables combination with high output. F = AB + BC + AC.

CHECKING;

By Idempotence law, we know that

([ABC + ABC)] + ABC) = (ABC + ABC) = ABC

Now the function F = A’BC + AB’C + ABC ‘ + ABC

= A’BC + AB’C + ABC’ + ([ABC + ABC)] + ABC)

= (ABC + ABC ‘) + (ABC + AB’C) + (ABC + A’BC)

= AB (C + C ‘) + A (B + B’) C + (A + A’) BC

= AB + BC + AC.

2. PRODUTS OF SUM (POS);

The product of sums form is a method (or form) of simplifying the Boolean expressions of logic gates. In this POS form, all the variables are ORed, i.e. written as sums to form sum terms.

All these sum terms are ANDed (multiplied) together to get the product-of-sum form. This form is exactly opposite to the SOP form. So this can also be said as “Dual of SOP form”.

Here the sum terms are defined by using the OR operation and the product term is defined by using AND operation. When two or more sum terms are multiplied by a Boolean OR operation, the resultant output expression will be in the form of product-of-sums form or POS form.

The product-of-sums form is also called as Conjunctive Normal Form as the sum terms are ANDed together and Conjunction operation is logical AND. Product-of-sums form is also called as Standard POS.

EXAMPLES;

(A+B) * (A + B + C) * (C +D)

(A+B) ̅ * (C + D + E ̅)

POS form can be obtained by

• Writing an OR term for each input combination, which produces LOW output.
• Writing the input variables if the value is 0, and write the complement of the variable if its value is 1.
• AND the OR terms to obtain the output function.

Ex: Boolean expression for majority function F = (A + B + C) (A + B + C ‘) (A + B’ + C) (A’ + B + C)

TRUTH TABLE;

Now write the input variables combination with high output. F = AB + BC + AC.

CHECKING;

        By Idempotence law, we know that

[(A + B + C) (A + B + C)] (A + B + C) = [(A + B + C)] (A + B + C) = (A + B + C)

Now the function

F = (A + B) (B + C) (A + C)

= (A + B + C) (A + B + C ‘) (A + B’ + C) (A’ + B + C)

= [(A + B + C) (A + B + C)] (A + B + C) (A + B + C ‘) (A + B’ + C) (A’ + B + C)

= [(A + B + C) (A + B + C ‘)] [(A + B + C) (A’ + B + C)] [(A + B + C) (A + B’ + C)]

= [(A + B) + (C * C ‘)] [(B + C) + (A * A’)] [(A + C) + (B * B’)]

= [(A + B) + 0] [(B + C) + 0] [(A + C) + 0] = (A + B) (B + C) (A + C)

                    MORE DETAILS;

           https://youtu.be/q5zta5kyru4

                                OR